

The one on left is based on the displacement of 300 units, while the one on the right shows the correct units. This is evident by noting that the lower tail for Brand B is at 0 instead of -300 (and that cell G6 contains 0 instead of -300).

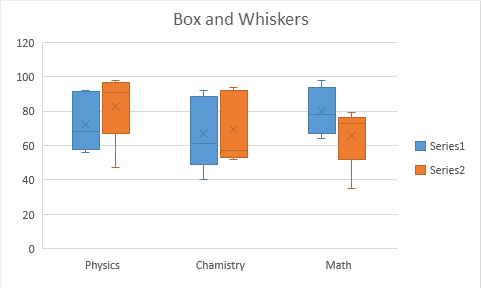
The key difference is that since the smallest data value is -300 (the value in cell F13), all the box plot values are shifted up by 300. The procedure is the same as for Example 1, except that this time we check the Use exclusive version of quartile option. Thus if R1 ranges from -10 to 20, the range in the chart will range from 0 to 30.Įxample 2: Create the box plot for the data in Figure 5.9.1 where cell B11 is changed to -300 and the exclusive version of the quartile function. Here, R1 is the data range containing the data. When a data set has one or more negative values, the y-axis will be shifted upward by the amount of -MIN(R1). We can also convert the box plot to a horizontal representation of the data (as shown in Figure 4) by first deleting the markers for the means (by clicking on any of these markers and pressing the backspace key) and then clicking on the chart and selecting Insert > Charts|Bar > Stacked Bar.įigure 4 – Horizontal Box Plot Box Plot with Negative Data Values Because of the long upper whisker (especially with respect to the box), Brand B may have an outlier (see Outliers and Robustness for a discussion of outliers).Īnother indication of symmetry is whether the × marker for the mean coincides with the median. We also see that the distribution of Brand A is pretty symmetric at least in the range between the 1 st and 3 rd quartiles, although there is some asymmetry for higher values (or potentially there is an outlier). See Ranking Functions in Excel for more details about the difference between these two versions.įrom the box plot in Figure 2, we can see that the scores for Brand C tend to be higher than for the other brands and those for Brand B tend to be lower.

If checked then the QUARTILE.EXC version of the 25 th and 75 th percentile is used (or QUARTILE_EXC for Excel 2007 users), while if this field is unchecked then the QUARTILE.INC (or equivalently the QUARTILE) version is used. There are two versions of this table, depending on whether or not you check or uncheck the Use exclusive version of quartile field. In particular, the meaning of each element in the box plot is described in Figure 3. The box itself is divided into two parts. For those who are interested, this table contains the information in Figure 3, as explained further in Special Charting Capabilities.įor each sample, the box plot consists of a rectangular box with one line extending upward and another extending downward (usually called whiskers). Note too that the data analysis tool also generates a table, which may be located behind the chart. The resulting chart is shown in Figure 2. Select the Box Plot option and insert A3:C13 in the Input Range. Check Headings included with the data and uncheck Use exclusive version of quartile. A dialog box will now appear as shown in Figure 4 of Descriptive Statistics Tools. To generate the box plots for these three groups, press Ctrl-m and select the Descriptive Statistics and Normality data analysis tool. Figure 1 summarizes the questionnaire scores from these groups. The 30 people are divided at random into 3 groups of 10 people each, where the first group evaluates Brand A, the second evaluates Brand B and the third evaluates Brand C.
CREATE BOX AND WHISKER PLOT EXCEL HOW TO
To generate a box plot, you can use the Box Plot option of the Descriptive Statistics and Normality data analysis tool found in the Real Statistics Resource Pack, as described in the following example. See also Special Charting Capabilities for how to create the box plot manually using Excel’s charting capabilities.Įxample 1: A market research company asks 30 people to evaluate three brands of tablet computers using a questionnaire. Specifically, a box plot provides a pictorial representation of the following statistics: maximum, 75 th percentile, median (50 th percentile), mean, 25 th percentile and minimum.īox plots are especially useful when comparing samples and testing whether data is distributed symmetrically. Another way to characterize a distribution or a sample is via a box plot (aka a box and whiskers plot).


 0 kommentar(er)
0 kommentar(er)
